Electrochemistry
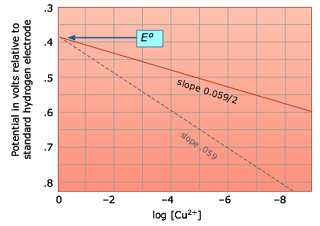The Nernst equation tells us that a half-cell potential will change by 59 millivolts per 10-fold change in the concentration of a substance involved in a one-electron oxidation or reduction; for two-electron processes, the variation will be 28 millivolts per decade concentration change. Thus for the dissolution of metallic copper
- Standard Half-cell Potentials
Problem Example 1 Find the standard potential of the cell Cu(s) | Cu2+ || Cl– | AgCl(s) | Ag(s)and predict the direction of electron flow when the two electrodes are connected. Solution: The net reaction corresponding to this cell will be 2 Ag(s) +...
- Transport Of Charge Within The Cell
For the cell to operate, not only must there be an external electrical circuit between the two electrodes, but the two electrolytes (the solutions) must be in contact. The need for this can be understood by considering what would...
- How To Balance Ionic Equations In Alkaline Medium?
(I) Standard Electrode Potential What information can we obtain from SEP? Sign – oxidation or reduction favoured Magnitude – the extent the reaction is favoured How to measure SEP? - connecting the redox couple (half cell) to SHE. - learn to draw...
- Electrochemistry : 10.1 Galvanic Cell (continued)
SPONTANEOUS REACTION occurs as the result of different ability of metal to give up their electron to flow through the circuit. CELL POTENTIAL (ECell) different in electrical potential of electrodes also called voltage or electromotive...
- Electrochemistry : 10.1 Galvanic Cell
Electrochemistry Study of relationship between chemical change & electric work Oxidation Loss of electron by species accompanied byn an increase in oxidation number Ex: Reduction Gain electron by a species accompanied by a decrease number...
Electrochemistry
Significance of the Nernst Equation.
Cu(s) → Cu2+ + 2e–
the potential E = (– 0.337) – .0295 log [Cu2+]
becomes more positive (the reaction has a greater tendency to take place) as the cupric ion concentration decreases. This, of course, is exactly what the Le Châtelier Principle predicts; the more dilute the product, the greater the extent of the reaction. Electrodes with poise
The equation just above for the Cu/Cu2+ half-cell raises an interesting question: suppose you immerse a piece of copper in a solution of pure water. With Q = [Cu2+] = 0, the potential difference between the electrode and the solution should be infinite! Are you in danger of being electrocuted? You need not worry; without any electron transfer, there is no charge to zap you with. Of course it won't be very long before some Cu2+ ions appear in the solution, and if there are only a few such ions per liter, the potential reduces to only about 20 volts. More to the point, however, the system is so far from equilibrium (for example, there are not enough ions to populate the electric double layer) that the Nernst equation doesn't really give meaningful results. Such an electrode is said to be unpoised. What ionic concentration is needed to poise an electrode? I don't really know, but I would be suspicious of anything much below 10–6 M.The Nernst equation works only in dilute ionic solutions
Ions of opposite charge tend to associate into loosely-bound ion pairs in more concentrated solutions, thus reducing the number of ions that are free to donate or accept electrons at an electrode. For this reason, the Nernst equation cannot accurately predict half-cell potentials for solutions in which the total ionic concentration exceeds about 10–3 M.- Standard Half-cell Potentials
Problem Example 1 Find the standard potential of the cell Cu(s) | Cu2+ || Cl– | AgCl(s) | Ag(s)and predict the direction of electron flow when the two electrodes are connected. Solution: The net reaction corresponding to this cell will be 2 Ag(s) +...
- Transport Of Charge Within The Cell
For the cell to operate, not only must there be an external electrical circuit between the two electrodes, but the two electrolytes (the solutions) must be in contact. The need for this can be understood by considering what would...
- How To Balance Ionic Equations In Alkaline Medium?
(I) Standard Electrode Potential What information can we obtain from SEP? Sign – oxidation or reduction favoured Magnitude – the extent the reaction is favoured How to measure SEP? - connecting the redox couple (half cell) to SHE. - learn to draw...
- Electrochemistry : 10.1 Galvanic Cell (continued)
SPONTANEOUS REACTION occurs as the result of different ability of metal to give up their electron to flow through the circuit. CELL POTENTIAL (ECell) different in electrical potential of electrodes also called voltage or electromotive...
- Electrochemistry : 10.1 Galvanic Cell
Electrochemistry Study of relationship between chemical change & electric work Oxidation Loss of electron by species accompanied byn an increase in oxidation number Ex: Reduction Gain electron by a species accompanied by a decrease number...